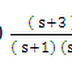Friday, 29 March 2013
Monday, 25 March 2013
Sunday, 24 March 2013
Saturday, 23 March 2013
Friday, 22 March 2013
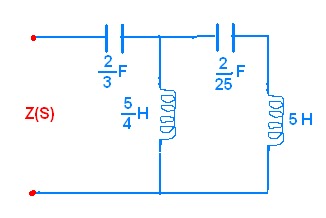
FOSTER FORM 1
It is used to realize impedance function.
Friday, 22 March 2013 by Unknown · 0
Thursday, 21 March 2013
PROPERTIES OF RC IMPEDANCE OR RL ADMITTANCE
Thursday, 21 March 2013 by Unknown · 0
Tuesday, 19 March 2013
Tuesday, 19 March 2013 by Unknown · 0
Sunday, 17 March 2013
1 INTRODUCTION TO SEMICONDUCTORS
2.BANDS,ELECTRON,HOLE
3.TYPES OF SEMICONDUCTORS
4.MASS ACTION LAW,MINORITY CARRIER CONCENTRATION
5.MOBILITY AND CONDUCTIVITY
6.CARRIER TRANSPORT PHENOMENUN
7.HALL EFFECT
8.EFFECT OF TEMPERATURE ON MOBILITY
9.CONTINUITY EQUATION
Sunday, 17 March 2013 by Unknown · 0
TO READ THE SPECIFIC TOPIC CLICK ON THE LINK GIVEN BY THE SIDE OF EACH TOPIC
INTRODUCTION TO DVMs
DIGITAL READOUTS OF DVMs
TYPES OF DVMs
RAMP TYPE
DUAL SLOPE INTEGRATING TYPE
by Unknown · 0
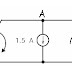
Ground comparator or zero level detector .so when the VR crosses zero and moves to negative values, the input at inverting terminal is greater than non inverting terminal. So output of this comparator produces a negative going pulse which makes the gating circuit to stop. Therefore the reading displayed on the display is the unknown voltage.
Where n is proportional to | VM | because the number of pulses depends upon VM
by Unknown · 0
Saturday, 16 March 2013
The sinusoidal waveform of figure 1 with its additional notation will now be used as a model in defining a few basic terms. These terms, however, can be applied to any alternating waveform. It is important to remember as you proceed through the various definitions that the vertical scaling is in volts or amperes and the horizontal scaling is always in units of time.
Saturday, 16 March 2013 by Unknown · 0
Number of digits
|
Maximum value
|
|
9/10
|
0,1,2,3………9
|
9
|
1/2
|
0,1
|
1
|
3/4
|
0,1,2,3
|
3
|
by Unknown · 0